The diffuse attenuation coefficient (abbreviated K_d(λ)) quantifies the rate of decrease of sunlight underwater in a narrow spectral band. It is often calculated from vertical profiles of irradiance; this rate of decrease is typically logarithmic.

One of the most common descriptors of the penetration of sunlight in water is the diffuse attenuation coefficient, K(λ), or K_d(λ) when calculated from vertical profiles of downwelling irradiance, E_d(λ). Where z is depth, this relationship is:

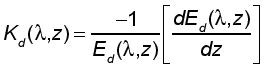
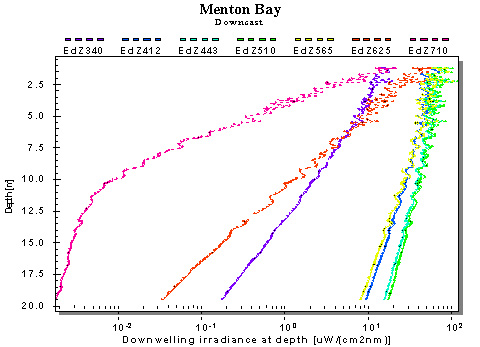
For homogeneous waters, a plot of the logarithm of E_d(λ,z) versus z forms a straight line, and K_d(λ,z) is the localized slope of that line (Figure 1).

The equation defining K_d can also be written as Ln[E_d(λ,z)/E_d(λ,z+dz)]=K_d(z)

This is a linear equation of the form Y=mx  where m=K_d is the slope.

When calculating K_d from vertical profiles of irradiance, irradiance values do not have to be calibrated as the irradiance units drop out.