Measurements during the 2017 total solar eclipse
Observations of solar radiation during a total solar eclipse present a rare opportunity for testing computer models that simulate the transfer of radiation through the atmosphere. Whereas a simple one-dimensional radiative transfer model with reduced solar irradiance at the top of the atmosphere can be used to calculate the brightness during partial eclipses with good accuracy, a much more sophisticated three-dimensional model is required to compute the spectral irradiance of the diffuse radiation at Earth's surface during a total eclipse (Emde and Mayer, 2007).
A total solar eclipse could be observed on August 21, 2017 across the United States, from Oregon in the West to South Carolina in the East. Germar Bernhard of BSI travelled to Smith Rock State Park (Figure 1), located in Oregon between Bend and Madras, to perform measurements with BSI's new GUVis-3511 multi-filter radiometer. Data collected during this campaign could potentially be used for the comparison with model calculations, such as those by Emde and Mayer (2007).
Smith Rock State Park is known as the birthplace of U.S. sport climbing and offered a specular backdrop for watching the celestial spectacle. The instrument was set up at 44.362950°N and 121,139667°W, 37 km (23 mi) south of the centerline of the moon's shadow, in a field sufficiently away from mountains to minimize the effect of obstructions to the horizon (Figure 2). (Despite the apparent close proximity of the park's mountains, rocks and trees in the vicinity of the instrument reduced the (cosine-weighted) diffuse irradiance only by about 1% according to our calculations.)
The partial eclipse started at 9:06:29 local time (PDT) and ended at 11:41:03. Totality occurred for one minute and 29 seconds, between 10:19:42 and 10:21:11.
The GUVis-3511 radiometer had 19 channels with wavelengths between 305 and 1,020 nm, and was equipped with a BioShade shadowband and BioGPS GPS receiver. It was set up on a sturdy tripod (Fig. 3) and powered by a 12 V dry-cell car battery and a sine-wave inverter.
The software was set up to perform one shadowband sweep every two minutes, between 7:36 and 12:30 PDT. Within each two-minute window, this configuration resulted in 45 seconds of global spectral irradiance measurements (with the shadowband stowed below the instrument's collector) and 75 seconds of data where the sky was partially blocked by the shadowband. The direct spectral irradiance was derived from these shadowband data with an algorithm developed for the BioShade accessory (Hooker et al., 2012). The aerosol optical depth was calculated from direct spectral irradiance at times when the moon was not blocking the Sun.
Results
Global Spectral Irradiance
Fig. 4 shows measurements of global (Sun + sky) spectral irradiance with photos overlaid over the graph at interesting times. There was some variability in the measurements before 9:30 and after 11:50 due to thin cirrus clouds and variable aerosols. During most of the eclipse, the sky was clear with little cloud and aerosol influence as indicated by the smoothly varying irradiance.
The photo taken at 10:18 shows the scene about one minute before the start of totality. Even though only a tiny part of the Sun was still visible at this time, the Sun still appears round in the photo because it is greatly overexposed. Note that the sky has color with yellow hues close to the trees.
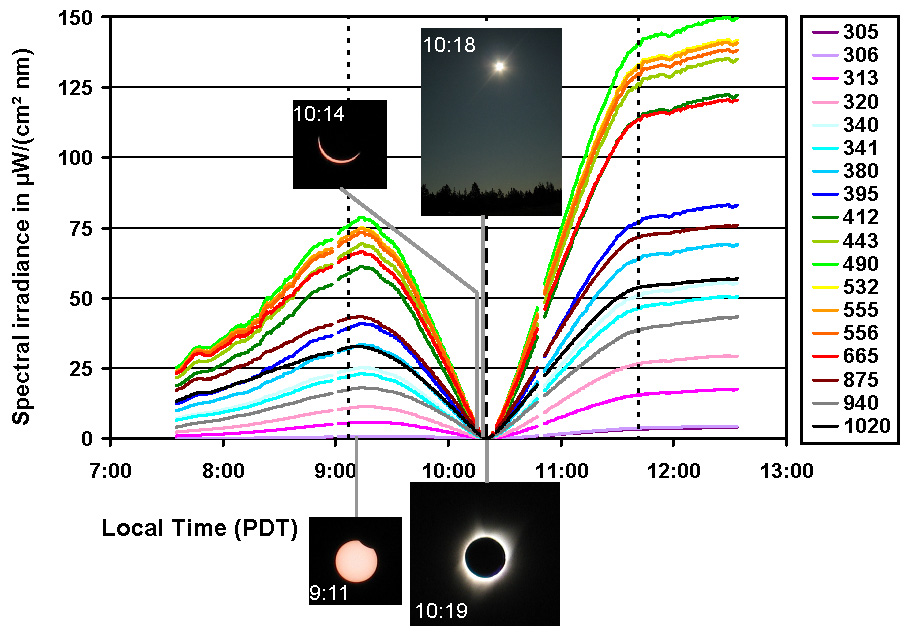
Short gaps at 9:00, 10:24, and 10:50 are due to dark measurements when the instrument's collector was covered. These measurements are important for accurate calculations of the instrument's net signal during the period of totality when the UV channels were close to the detection limit. All data have been corrected for the cosine error of the instrument's collector using laboratory measurements of the collector's directional response and the ratio of direct and diffuse irradiance calculated from the shadowbanding segments.
Note that four of the instrument's 19 channels have wavelengths normally not used in BSI's instruments: 306, 341, 556, and 940 nm. The three shorter wavelengths are experimental channels for testing a new type of photodiode while the 940 nm channel will be used in the future for water vapor retrievals.
Fig. 5a shows the data of Figure 4 plotted on a logarithmic vertical axis. At the time of totality, spectral irradiance at all wavelengths is about 4 - 5 orders of magnitude lower compared to values when the Sun is not obstructed. Figure 5b shows only measurements five minutes before and after the period of totality, also on a logarithmic axis. There are gaps in the plot when the instrument was shadowbanding, and because of this, there are no global irradiance data available for the later part of totality.
Note that spectral irradiances at long wavelengths (e.g., 1020 nm) drop must faster than those at shorter wavelengths (e.g., 490 nm) immediately before the period of totality (first dashed-dotted vertical line in Figure 5b). This phenomenon can be easily explained: radiation at longer wavelengths has a larger direct component than radiation at shorter wavelengths, which is more diffuse and has entered the atmosphere outside the moon's shadow. When entering totality, longer wavelengths are therefore more efficiently blocked.
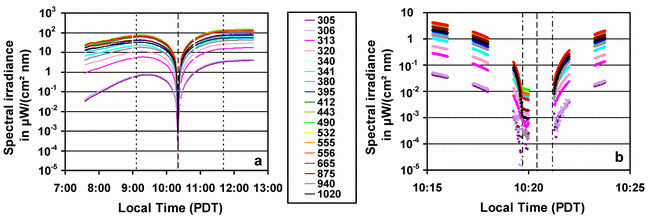
Spectral irradiances at UV and visible wavelengths also show interesting variations over the course of the eclipse. To explain these variations quantitatively, it has to be taken into account that the Sun is not uniformly bright across its disk. Instead, it is darker at the edge than in the center (called "solar limb darkening"), and this variation also depends on wavelength. Close to or within the period of totality, three-dimensional radiative transfer modeling is required for accurate calculations of the diffuse irradiance.
Validation with Radiative Transfer Modeling
While a three-dimensional radiative transfer (RT) model is required to accurately simulate the measurements during the period of totality, a one-dimensional RT model is adequate for validating GUVis-3511 measurements before or after the event, and during most of the partial eclipse period. (A one-dimensional model assumes that the composition of the atmosphere only varies in vertical direction and that the Earth is homogenously illuminated by the Sun without the moon casting a shadow anywhere.)
Model calculations were performed with the RT model UVSPEC/libRadtran (Mayer and Kylling, 2005). Aerosol extinction was parameterized with the AODs derived from the shadowband measurements). Total ozone was calculated from global spectral measurements at 313 and 340 nm using a look-up table.
Figure 6 compares direct (red) and global (blue) measurements with the model calculations for 12:00 PDT, about 20 minutes after the end of the eclipse. Measurement and model are in agreement to within a few percent (i.e., to within their respective uncertainties), confirming the good quality of the GUVis-3511 measurements.

Concluding remarks
A more in-depth analysis of the results discussed above were published in Atmospheric Chemistry and Physics (Bernhard and Petkov, 2018 and Ockenfuß et al., 2020). The paper by Ockenfuß et al. (2020) compares GUV measurements performed during totality with 3-D Monte Carlo radiative transfer calculations.
References
Bernhard, G. and P. Petkov. (2019). Measurements of spectral irradiance during the solar eclipse of 21 August 2017: reassessment of the effect of solar limb darkening and of changes in total ozone, Atmospheric Chemistry and Physics, 19, 4703-4719. The paper is available here.
Emde, C. and Mayer, B. (2007) Simulation of solar radiation during a total eclipse: a challenge for radiative transfer. Atmos. Chem. Phys., 7(9), 2259-2270.
Hooker, S. B., Bernhard, G., Morrow, J. H., Booth, C. R., Comer, T., Lind, R. N., and Quang, V. (2012). Optical Sensors for Planetary Radiant Energy (OSPREy): calibration and Validation of Current and Next-Generation NASA Missions., NASA Goddard Space Flight Center, NASA/TM–2011–215872, https://ntrs.nasa.gov/search.jsp?R=20130003503.
Mayer, B. and Kylling, A. (2005) Technical Note: The libRadtran software package for radiative transfer calculations: Description and examples of use, Atmos. Chem. Phys., 5, 1855–1877, https://www.atmos-chem-phys.net/5/1855/2005/.
Ockenfuß, P., C Emde, B. Mayer, and G. Bernhard. (2020). Accurate 3-D radiative transfer simulation of spectral solar irradiance during the total solar eclipse of 21 August 2017, Atmos. Chem. Phys., 20, 1961–1976. The paper is available here.



